Navigating The World Of Maps: Understanding And Solving Scale Math Problems

Navigating the World of Maps: Understanding and Solving Scale Math Problems

Related Articles: Navigating the World of Maps: Understanding and Solving Scale Math Problems

Introduction

With great pleasure, we will explore the intriguing topic related to Navigating the World of Maps: Understanding and Solving Scale Math Problems. Let’s weave interesting information and offer fresh perspectives to the readers.

Navigating the World of Maps: Understanding and Solving Scale Math Problems

Maps are essential tools for navigation, exploration, and understanding our world. They provide a simplified representation of geographical features and distances, allowing us to visualize and comprehend vast areas at a glance. However, the accuracy and usefulness of maps rely heavily on the concept of scale. Map scale represents the ratio between the distance on a map and the corresponding distance on the ground. This relationship forms the foundation for a wide range of calculations, often referred to as map scale math problems.

Understanding the Fundamentals of Map Scale

Map scale is typically expressed in three common ways:

• Verbal Scale: This method uses words to describe the relationship between map distance and ground distance. For example, "1 centimeter on the map represents 1 kilometer on the ground."
• Representative Fraction (RF): This method expresses the scale as a fraction, where the numerator represents the map distance and the denominator represents the corresponding ground distance. For example, 1:100,000 means that one unit on the map represents 100,000 units on the ground.
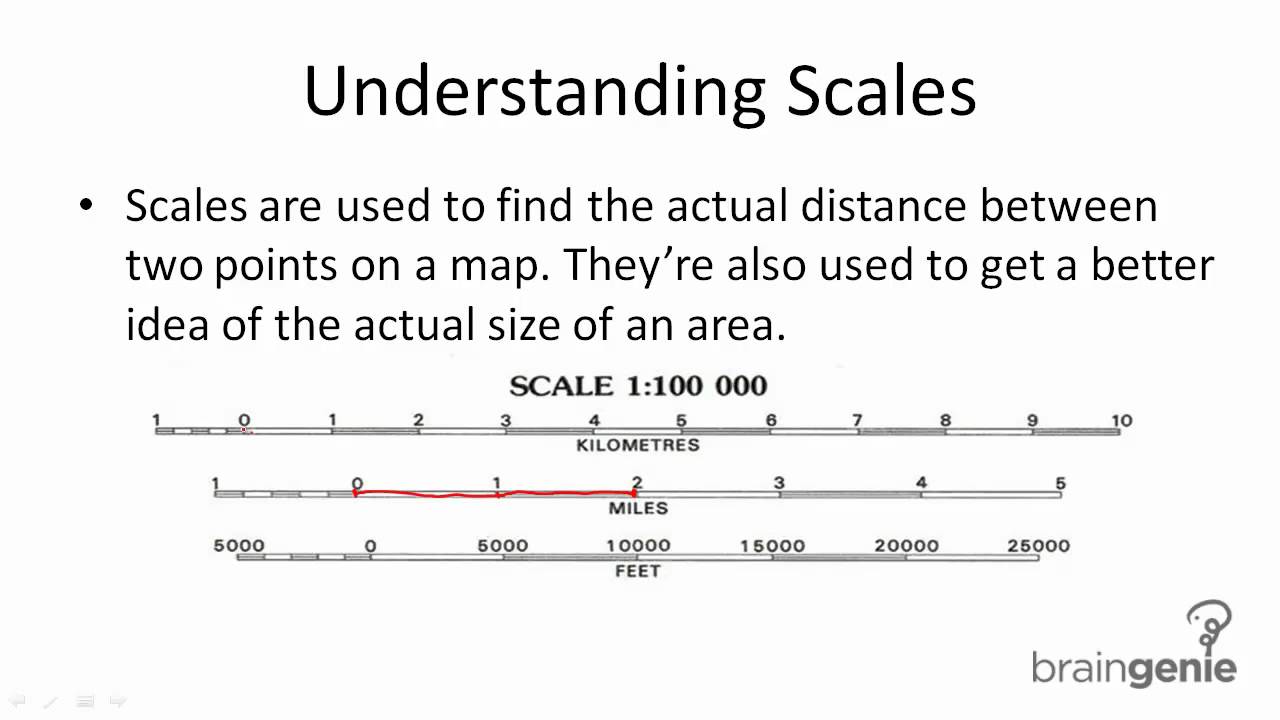
• Graphic Scale: This method uses a visual representation of the scale, typically a bar divided into segments representing specific distances on the ground. This allows for quick and visual estimations of distances on the map.

The Importance of Map Scale in Problem-Solving

Map scale math problems are essential for various applications, including:

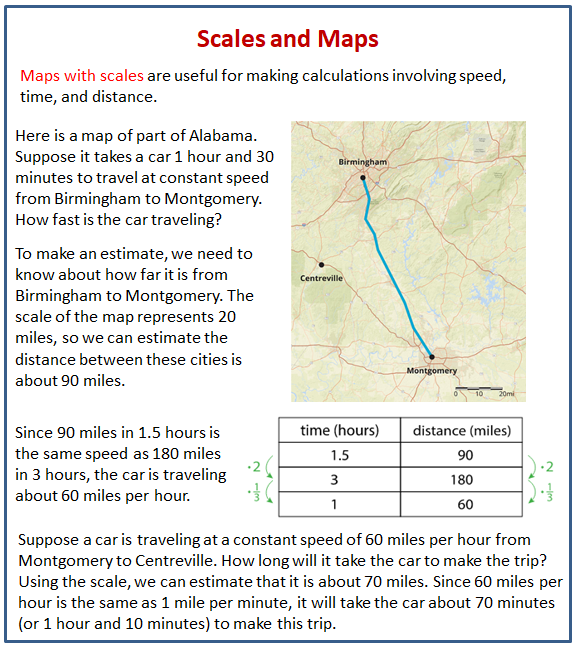
• Calculating Actual Distances: By using the map scale, individuals can determine the actual distance between two points on the ground based on the measured distance on the map. This is crucial for planning journeys, estimating travel time, and understanding the geographical extent of features.
• Determining Map Distances: Conversely, knowing the actual distance between two points allows us to calculate the corresponding distance on the map using the scale. This is helpful for creating accurate maps or for understanding the relative sizes of features depicted on a map.
• Estimating Areas: Map scale plays a crucial role in calculating the area of a region depicted on a map. By applying the scale to the measured area on the map, one can determine the actual area of the corresponding region on the ground. This is essential for tasks such as land management, resource planning, and environmental analysis.
• Understanding Map Projections: Map scale is closely related to the concept of map projections. Different projections distort distances and shapes in varying degrees, and understanding the scale of a map is vital for interpreting the accuracy and limitations of the projection used.

Types of Map Scale Math Problems

Map scale math problems can be categorized into various types, each requiring specific approaches and calculations:

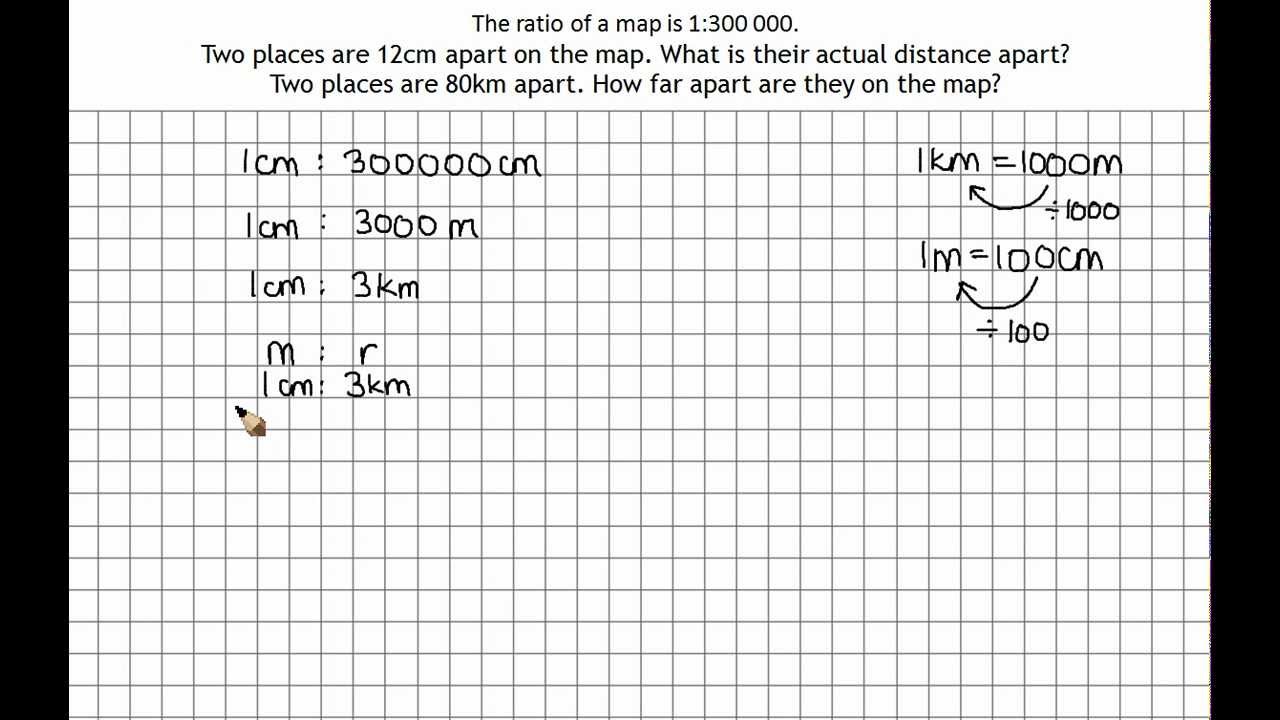
• Direct Proportion Problems: These problems involve directly applying the scale to determine either the ground distance or the map distance. For example, if the scale is 1:50,000, and the distance on the map is 5 cm, the actual ground distance would be 5 cm x 50,000 = 250,000 cm or 2.5 km.
• Conversion Problems: These problems involve converting distances between different units of measurement. For instance, if the scale is 1:250,000 and the distance on the map is 3 cm, the actual distance needs to be converted from centimeters to kilometers.
• Area Calculation Problems: These problems involve calculating the area of a region on the ground using the scale and the measured area on the map. This often involves converting units of measurement and applying appropriate formulas for calculating area.
• Scale Conversion Problems: These problems involve converting between different scales, such as from a verbal scale to a representative fraction or from a graphic scale to a verbal scale. This requires understanding the relationships between different scale representations.

Solving Map Scale Math Problems: A Step-by-Step Approach

Solving map scale math problems typically involves the following steps:

1. Identify the Scale: Determine the scale of the map, whether it is expressed verbally, as a representative fraction, or graphically.
2. Convert Units: Ensure that the units of measurement for the map distance and the ground distance are consistent. If necessary, convert between different units, such as centimeters to kilometers or inches to miles.
3. Apply the Scale: Use the scale to calculate the unknown distance, either on the map or on the ground, based on the known distance.
4. Verify and Interpret: Review the calculated answer to ensure it is logical and consistent with the scale and the context of the problem.

Examples of Map Scale Math Problems

Let’s illustrate these concepts with some practical examples:

Example 1: Calculating Ground Distance

A map has a scale of 1:50,000. The distance between two towns on the map is 7 cm. What is the actual distance between the towns?

Solution:

• The scale 1:50,000 means that 1 cm on the map represents 50,000 cm on the ground.
• Therefore, 7 cm on the map represents 7 cm x 50,000 = 350,000 cm on the ground.
• Converting centimeters to kilometers, we get 350,000 cm / 100,000 cm/km = 3.5 km.

Example 2: Calculating Map Distance

The actual distance between two cities is 120 km. The map scale is 1:1,000,000. What is the distance between the cities on the map?

Solution:

• The scale 1:1,000,000 means that 1 cm on the map represents 1,000,000 cm on the ground.
• Converting kilometers to centimeters, we get 120 km x 100,000 cm/km = 12,000,000 cm.
• Therefore, the distance between the cities on the map is 12,000,000 cm / 1,000,000 = 12 cm.

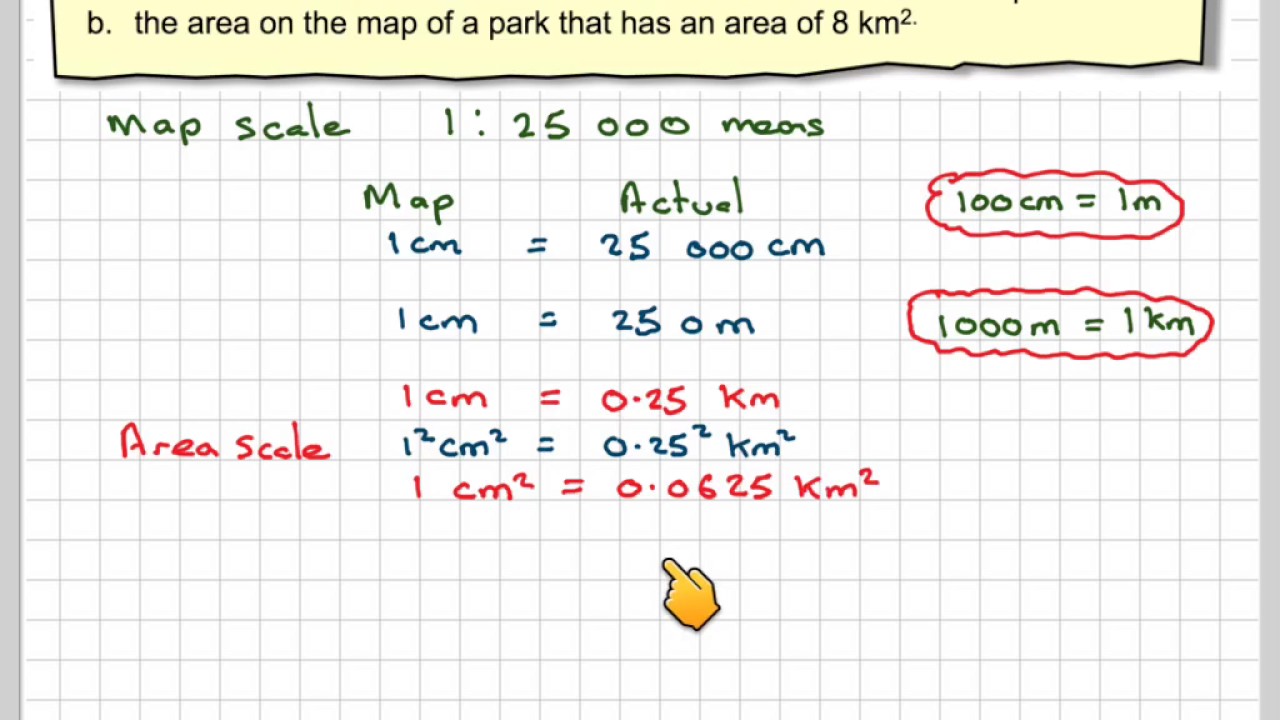
Example 3: Calculating Area

A rectangular park on a map with a scale of 1:25,000 measures 4 cm by 6 cm. Calculate the actual area of the park.

Solution:

• The area of the park on the map is 4 cm x 6 cm = 24 cm².
• The scale 1:25,000 means that 1 cm on the map represents 25,000 cm on the ground.
• Therefore, 1 cm² on the map represents 25,000 cm x 25,000 cm = 625,000,000 cm² on the ground.
• The actual area of the park is 24 cm² x 625,000,000 cm²/cm² = 15,000,000,000 cm² or 15,000,000 m².

FAQs about Map Scale Math Problems

Q: What is the difference between a large scale and a small scale map?

A: A large-scale map represents a smaller area with more detail, while a small-scale map represents a larger area with less detail. The representative fraction for a large-scale map will have a smaller denominator, indicating that a smaller distance on the map represents a larger distance on the ground.

Q: Can I use a map with a different scale to solve a problem?

A: It is generally not recommended to use a map with a different scale to solve a problem. The scale is crucial for accurate calculations, and using a different scale can lead to significant errors.

Q: How do I convert between different units of measurement in map scale problems?

A: You can use conversion factors to convert between different units of measurement. For example, to convert centimeters to kilometers, you would divide by 100,000 (1 km = 100,000 cm).

Q: What are some common mistakes to avoid when solving map scale math problems?

A: Common mistakes include:

• Not understanding the scale and its relationship to the distances on the map and the ground.
• Not converting units of measurement consistently.
• Misinterpreting the scale or using the wrong formula for calculations.

Tips for Solving Map Scale Math Problems

• Read the Problem Carefully: Understand the information provided and the question being asked.
• Identify the Scale: Determine the scale of the map and ensure it is appropriate for the problem.
• Convert Units: Make sure all distances are in the same units before performing calculations.
• Use the Correct Formula: Apply the appropriate formulas for calculating distances, areas, or scale conversions.
• Check Your Answer: Review your calculations and ensure the answer is logical and consistent with the scale.

Conclusion

Map scale math problems are an essential part of understanding and utilizing maps effectively. By mastering the concepts of scale, units of measurement, and problem-solving techniques, individuals can confidently navigate and interpret geographical information. Whether planning a journey, analyzing environmental data, or simply appreciating the world around us, understanding map scale empowers us to extract valuable insights from these powerful tools of representation.

Closure

Thus, we hope this article has provided valuable insights into Navigating the World of Maps: Understanding and Solving Scale Math Problems. We thank you for taking the time to read this article. See you in our next article!